Perimeter and Area: From Activities to Assessments
There is computational fluency and there is conceptual understanding in mathematics. A false dichotomy between fluency and understanding is common among criticisms against how students are taught math. It is true that procedural competence in math does not guarantee a deeper understanding, but one must not jump at the conclusion that the lack of understanding in math is due to an overemphasis on arithmetic competence. Oftentimes, the problem is not a lack of conceptual understanding but the presence of misconceptions. A misunderstanding of concepts in math can happen when the teaching of concepts themselves is not well designed. These problems can arise from two sides, activities (how math is taught) and assessments (how knowledge and skills are gauged).
Perimeter and area are good examples.
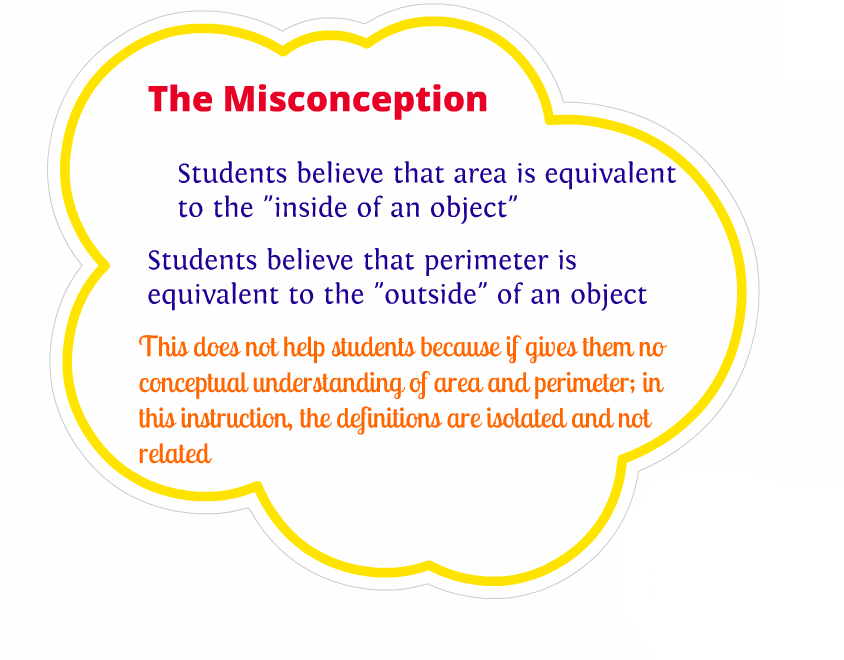
Eric Horn illustrates this in the following slides:
The part, "Do we use area or perimeter", can either be a learning activity or an assessment opportunity. It is an excellent activity because it opens the eyes of the students to applications. As an assessment, it is questionable. How much carpet is easily associated with area but paint is not as straightforward. Paint is measured in volume and how much paint is required really depends on how many coats of paint are going to be applied on the wall.
We had our basement recently renovated so my son saw the work involved when we had new flooring installed. So I asked him what I needed to know about a room in case I want to estimate how many tiles I need to change the flooring. And my son knew it was the area. So I added, "For the baseboard moulding (while specifically pointing out to him in our bedroom what a base moulding is), what measurement do I need?" And he answered, "perimeter". Now, we did not discuss anything about the wall so I would not know at this time if my son has the misconception Eric Horn pointed out in the previous slides. But I think this is a proper assessment of whether my son does know the difference between area and perimeter.
My son's third grade class is currently covering measurements, perimeter and area. One of the activities he has explored is the following. It is a floor design:
My son is no architect at this point obviously. I still have to explain to him that a basement is not really a room. But aside from architectural challenges, I have circled on the upper right hand corner what the grid is. It is not one inch, but 1/2 inch. Thus, if I have to report the perimeter of the section my son marked as a "game room", I will write 11 inches and not 22. The area gets pretty tricky since it is (5 times 1/2 times 6 times 1/2) or 7 1/2 square inches. The section enclosed inside my son's "game room" consists of 30 squares but each of this square is 1/4 square inch, so that also amounts to 30/4 or 7 1/2 square inches. Personally, I do not know the pedagogical reason behind specifying a 1/2 inch grid. And this is not easily ignored when the perimeter is involved. On the other hand, a child is very likely to report the wrong area. In my son's picture, there are indeed 30 squares inside his "game room", but not 30 square inches. The above is certainly an excellent activity. However, it cannot serve as a good assessment since it is testing the child on other details apart from area and perimeter.
Achieving a conceptual understanding in math obviously depends on good activities. These activities can be tailored such that misconceptions are avoided. John Gough also lists several in a paper published in Prime Number. Here is one common misconception mentioned:
What is the area and perimeter of the shaded regions in the following:
Brynne could have easily provided the correct answers by just knowing how to count: All have the same perimeter (14 units), but the first one has an area of 16 squares, the second one has 15 squares, and the last one has 12 squares. Brynne can arrive at these correct responses by correctly executing the procedures of determining the perimeter and area without being aware that she currently has the misconception that one and only one value of area is possible for any given perimeter. As an assessment, it can fail in diagnosing a possible misconception. As an activity, the above exercise can be excellent especially if the teacher points out at the end that the above three nicely demonstrate that the area is not defined by perimeter. The area inside a fixed length loop depends on the shape one makes with that loop.
We focus too often on assessments and what these tell us about how children learn. What information assessments provide is frequently limited. On the other hand, there is sometimes too much reliance on discovery learning. Problem solving and activities can indeed provide opportunities for students to understand concepts more deeply. The fact that mathematical fluency is not a guarantee for conceptual understanding should make us realize that knowing procedures and practice are usually inadequate. Students do not usually discover what needs to be discovered. There are math activities that are designed with the right conditions like the one illustrated above (red squares). As an assessment tool, doing this activity correctly shows procedural competence but not necessarily a conceptual understanding. It only follows then that as an activity, direct instruction from the teacher regarding the underlying concept is still necessary.
In mathematics, the debate between rote learning and deeper understanding is not a helpful one. What is needed is a correct appreciation of what various activities and assessments entail so that children can develop both procedural competence as well as a conceptual understanding.
Perimeter and area are good examples.
Eric Horn illustrates this in the following slides:
The part, "Do we use area or perimeter", can either be a learning activity or an assessment opportunity. It is an excellent activity because it opens the eyes of the students to applications. As an assessment, it is questionable. How much carpet is easily associated with area but paint is not as straightforward. Paint is measured in volume and how much paint is required really depends on how many coats of paint are going to be applied on the wall.
We had our basement recently renovated so my son saw the work involved when we had new flooring installed. So I asked him what I needed to know about a room in case I want to estimate how many tiles I need to change the flooring. And my son knew it was the area. So I added, "For the baseboard moulding (while specifically pointing out to him in our bedroom what a base moulding is), what measurement do I need?" And he answered, "perimeter". Now, we did not discuss anything about the wall so I would not know at this time if my son has the misconception Eric Horn pointed out in the previous slides. But I think this is a proper assessment of whether my son does know the difference between area and perimeter.
My son's third grade class is currently covering measurements, perimeter and area. One of the activities he has explored is the following. It is a floor design:
My son is no architect at this point obviously. I still have to explain to him that a basement is not really a room. But aside from architectural challenges, I have circled on the upper right hand corner what the grid is. It is not one inch, but 1/2 inch. Thus, if I have to report the perimeter of the section my son marked as a "game room", I will write 11 inches and not 22. The area gets pretty tricky since it is (5 times 1/2 times 6 times 1/2) or 7 1/2 square inches. The section enclosed inside my son's "game room" consists of 30 squares but each of this square is 1/4 square inch, so that also amounts to 30/4 or 7 1/2 square inches. Personally, I do not know the pedagogical reason behind specifying a 1/2 inch grid. And this is not easily ignored when the perimeter is involved. On the other hand, a child is very likely to report the wrong area. In my son's picture, there are indeed 30 squares inside his "game room", but not 30 square inches. The above is certainly an excellent activity. However, it cannot serve as a good assessment since it is testing the child on other details apart from area and perimeter.
Achieving a conceptual understanding in math obviously depends on good activities. These activities can be tailored such that misconceptions are avoided. John Gough also lists several in a paper published in Prime Number. Here is one common misconception mentioned:
Brynne says that the area inside a fixed length loop of string is always the same because the length is always the same.It is easy to spot a misconception when a student fully describes the concept that the student thought to be correct. Unfortunately, it is not as straightforward to get this information from a poorly designed assessment. For instance, Brynne could have been given the following task:
What is the area and perimeter of the shaded regions in the following:
Brynne could have easily provided the correct answers by just knowing how to count: All have the same perimeter (14 units), but the first one has an area of 16 squares, the second one has 15 squares, and the last one has 12 squares. Brynne can arrive at these correct responses by correctly executing the procedures of determining the perimeter and area without being aware that she currently has the misconception that one and only one value of area is possible for any given perimeter. As an assessment, it can fail in diagnosing a possible misconception. As an activity, the above exercise can be excellent especially if the teacher points out at the end that the above three nicely demonstrate that the area is not defined by perimeter. The area inside a fixed length loop depends on the shape one makes with that loop.
We focus too often on assessments and what these tell us about how children learn. What information assessments provide is frequently limited. On the other hand, there is sometimes too much reliance on discovery learning. Problem solving and activities can indeed provide opportunities for students to understand concepts more deeply. The fact that mathematical fluency is not a guarantee for conceptual understanding should make us realize that knowing procedures and practice are usually inadequate. Students do not usually discover what needs to be discovered. There are math activities that are designed with the right conditions like the one illustrated above (red squares). As an assessment tool, doing this activity correctly shows procedural competence but not necessarily a conceptual understanding. It only follows then that as an activity, direct instruction from the teacher regarding the underlying concept is still necessary.
In mathematics, the debate between rote learning and deeper understanding is not a helpful one. What is needed is a correct appreciation of what various activities and assessments entail so that children can develop both procedural competence as well as a conceptual understanding.






Comments
Post a Comment